Theorem (Green-Tao) Let A be any subset of the prime numbers of positive relative upper density, thus

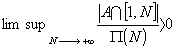
where 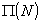denotes the number of primes less than or equal to N. Then A contains arithmetic progressions of length K for all K.

Specialization : Consider the set of primes p = 1 (mod 4)

Theorem : (classical) Every prime p = 1 (mod 4) can be written as the sum of two squares.

Quadratic corollary : There are arbitrarily long arithmetic progressions consisting of numbers which are the sum of two squares.

Special case : k = 4.

Theorem (Heath-Brown) Let S be the set of sums of two squares. There are infinitely many 4-term arithmetic progressions in S.

Proof : The numbers (n-1)²+(n-8)², (n-7)²+(n+4)², (n+7)²+(n-4)² and (n+1)²+(n+8)² form a 4-term arithmetic progression for any strictly positive integer.

Remark : n = 1 : 7², 6²+5², 8²+3², 2²+9² (Degenerated cases)
n = 4 : 3²+4², 3²+8², 11², 5²+12²
n = 7 : 6²+1², 11², 14²+3², 8²+15²
n = 8 : 7², 1²+12², 15²+4², 9²+17²