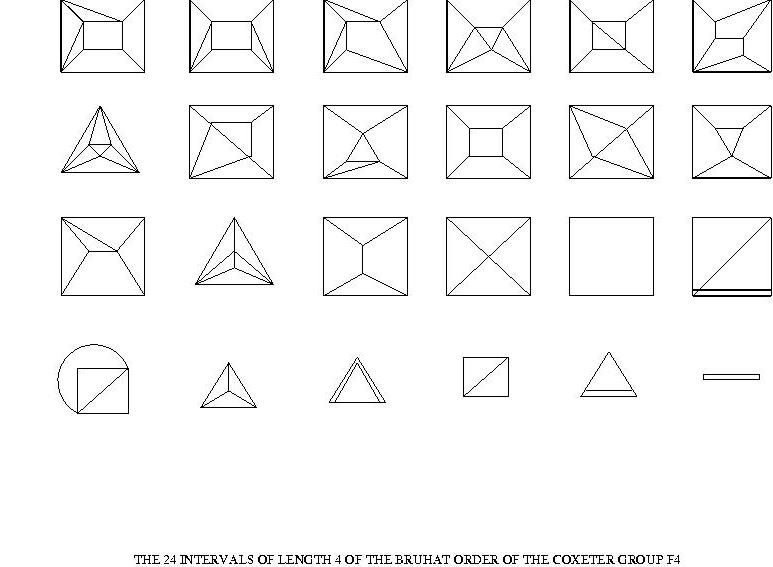
33148 - The 24 Intervals of length 4 of the Bruhat order of the Coxeter Group F4
N. Lygeros
F4 is one of the six exceptional groups of Coxeter E6, E7, E8, F4, H3 and H4. For finite Coxeter groups create also three one-parameter families of increasing rank An, Bn, Dn and one-parameter family of dimension two I2 (p). Coxeter classified them in 1935 with what we call now Coxeter-Dynkin diagrams.
F4 is described in this context as: F4 = [3,4,3]
Each node represents a mirror and he label attached to the branch encodes the dihedral angle order between two mirrors. The unlabeled branches implicitly represent the order 3.
The Bruhat order is a partial order on the group W where (W,S) is a Coxeter system with generators S.
The reduced word for an element w of W is a minimal length expression of w as a product of elements of S and the length l(w)of w is the length of a reduced word.
The Bruhat order is defined by u ≤ v if some substring of some reduced word for v is a reduced word for u.
F4 is a solvable group of order 1152.
The study Dyer’s conjecture on Coxeter groups, we compute all the intervals of length 4 of the Bruhat order of the Coxeter F4 and we find 24 up to isomorphy. At that point, it is possible to see a relation with the 24-cell with its regular net.